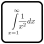Ice Cubes Sudoku
(Published on 1. July 2024, 02:39 by psams)
Rules
Standard Sudoku rules apply.
Give each cell in the grid an index number 1 to 81 in reading order, left to right, top to bottom. The blue marked cells may contain any digit 1-9. Each unmarked cell may only contain a digit 1-9 which appears in the mathematical cube of its index number. Zeroes are not used.
For example, 37 cubed = 50653 so digits 3, 5, and 6 may go in unmarked cell R5C1. 16 cubed = 4096, but R2C7 is a marked cell, so any digit may go there.
Values on a black dot are in a 1:2 ratio. Not all black dots are given.
Reference: Table of Cubes on calculatorsoup.com
Solution code: Row 5 Digits, Column 5 Digits
Comments
on 12. July 2024, 21:23 by ParaNox
I can see how you were excited about your discovery, which is indeed interesting.
But solving the grid this way felt more like a chore and then doing a pencilmark sudoku.
Please don't take this the wrong way, as at least I've learned something new about a 9x9 sudoku grid today, filling the cells with possible digits however did not feel like actually solving something or discovering logical steps myself, so basically half the fun of finding the break-in was lost.
Maybe setting this as a pencilmark sudoku or something similar would've been a more enjoyable alternative for me.
on 1. July 2024, 20:40 by psams
Archon, you lost me at Autohotkey and buffer-stuff, but I am thinking about another puzzle that will need to use excel to generate a set of digits. For this one, I imagine Simon trying to calculate all those cubes in his head, then giving up and spending a few minutes entering them all from a table, and then solving the puzzle in almost no time. Worst CtC ever.
on 1. July 2024, 20:12 by Archon
Have to admit, I used Excel to generate all the center-mark options and Autohotkey to buffer-stuff them. Still, got to flex my spreadsheet-making skills.:)
on 1. July 2024, 15:24 by psams
Also, I wanted to mention, my favorite cubes values from the table were 62^3 = 238328 and 68^3 = 314432 (which places 1234 in the cell). And no, neither is my ATM code.
on 1. July 2024, 14:45 by psams
Though the result is easy, I found the task of setting this puzzle to be challenging without using any solving software. But I was amazed at how far I was able to place digits in the grid with no conflicts at the start. Had I discovered an entire Sudoku in the wild? The first conflicts were in cells that began with 0's in them from the cubes table, so I treated them as "wild," and after that, filled over 1/3 of the puzzle before running into an unsolvable conflict in a non-zero filled cell at R4C7. After iterating on changes to fix the puzzle, of the final blue cells, only 5 did not initially come from cube values with 0's, and I had only 1 given, in cell R6C9. I did not want to reveal that given at the start, so others could experience the solve order as I had, so I used the Kropki dot to set the value instead (and thus keep it hidden until much else was solved.) I hope others will experience the sense of surprise that I did, and thus I did not prefill the grid with the numbers from the cubes table. For a much harder challenge, try setting your own variant from scratch, starting with no blue cells or Kropki, treating 0's as any digit, and overwriting a minimal number of the non-zero cells. Can you find a puzzle with less than 5 such cells and 1 given? Bonus stars for not using solving software.
on 1. July 2024, 12:09 by 1121
Easy puzzle with 11:40 solving time. I think longest part was entering candidates into cells.
on 1. July 2024, 03:03 by psams
Changed difficulty rating. It is not hard to solve. There is a clear deduction path from start to finish with just a couple of steps that may take a little time to see.