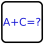Leftover Goodies - A 9x9 Remainder Sudoku
(Eingestellt am 6. April 2022, 15:13 Uhr von Playmaker6174)
It's been known that this variant was used several times in some competitions in the past and quite randomly, I thought about this variant while summarizing my ideas for that 6x6 pack and as recommendations from lots of people, I decided to finally make a 9x9 version. It's actually quite difficult to make a nice fun one without adding so many confusing clues in the grid so I hope you enjoy this final result here!
Rules:
- Normal Sudoku rules apply: every row, column and 3x3 box contains digits from 1 to 9 each once.
- A circle with a number in it that connects two (orthogonally adjacent) cells shows the remainder when the bigger digit is divided by the smaller digit.
For people not so familiar with how the rule works, this is the explanation:
Let two positive integers a and b such that a is greater than b, then there exists unique positive integer q and unique non-negative integer r such that r is smaller than b and this equation happens: a = b x q + r. By definition, q is called the quotient (result) and r is called the remainder.
In this puzzle, we only care about the remainder. For example, 9 and 2 will have 1 in the circle connected between them, since 9 divided by 2 is 4 as the result and 1 as the remainder (9 = 2 x 4 + 1).
Puzzle:
Good luck and have fun solving!
Lösungscode: Row 8 (left to right) and column 9 (top to bottom), 18 digits long with no space
Kommentare
am 26. April 2022, 01:14 Uhr von SimplePurpleFrog
Very good puzzle, interesting rules, some very clever logic, I loved the break-in.
am 12. April 2022, 12:30 Uhr von Piatato
Thanks for the great puzzle Playmaker6174! Hail the king of amazing solve paths!
am 10. April 2022, 08:46 Uhr von aruvi
very cool constraint, some unique logic in here
am 9. April 2022, 13:26 Uhr von grkles
Loved this variant!!!!
am 9. April 2022, 12:09 Uhr von kolot
How did I miss this puzzle? I was looking for a 9x9 version of this rule set. It was over there and I didn’t see it. Very nice implementation of the rule set. Very smooth and fun solve.
am 7. April 2022, 22:07 Uhr von the_cogito
Really cool puzzle, nicely done!
am 7. April 2022, 20:36 Uhr von Playmaker6174
Changed the CTC link because it's too hard to look
am 7. April 2022, 13:42 Uhr von Niverio
Very fun! there is a certain pair of digits that do SO MUCH work in this puzzle. Flows very nicely too.
---
Thank you for your lovely feedback Niv!
am 7. April 2022, 07:39 Uhr von Playmaker6174
Thanks to all of you Vebby, Jesper and DVFrank! I’m glad that y’all had a lot of fun and found it enjoyable :)
am 6. April 2022, 17:27 Uhr von DVFrank
Very nice! That's a lovely constraint with so much potential, although I did find it quite tricky to get a good feel for it at first :^)
am 6. April 2022, 16:48 Uhr von Jesper
Great fun, thanks!
am 6. April 2022, 16:11 Uhr von SKORP17
Ist Division durch 1 erlaubt ?
---
Yes it is.
am 6. April 2022, 15:19 Uhr von Vebby
Wonderful construction! Great exploration of the constraint. Had a lot of fun solving it :)