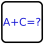Sudoku X6
(Eingestellt am 13. Juli 2018, 18:43 Uhr von DarkBeamIta)
Fill this puzzle with numbers 1 to 10 in each cell, but in colored cells you cannot insert any 10.
Colored cells can either be green or yellow, and form three four digit group (yellow) and two five digit groups (green), either aligned in a row or a column.
If you interpret each group as a number when you multiply each yellow number by 6 you get three numbers.
You read two of those numbers in the exact digit order in the green groups. The other resulting number can be obtained reading one of those two green digit groups in a different order, every digit has a different position though.
(No 4-digit coupled with another 4-digit has a same digit in a same position, all yellow numbers are odd.)
Lösungscode: ROWS B AND I
Kommentare
am 14. Juli 2018, 09:49 Uhr von DarkBeamIta
Added a clue so that now the puzzle has only one solution thanks zorant
am 13. Juli 2018, 21:50 Uhr von dm_litv
it's hard not to agree
am 13. Juli 2018, 21:38 Uhr von sf2l
agree with zoran
am 13. Juli 2018, 20:34 Uhr von DarkBeamIta
Odd is not divisible by 2. Y.n. is a yellow number :D
Please ask if you find other doubts... I am always in uncertainty about explanations!
am 13. Juli 2018, 20:23 Uhr von Luigi
No y.n. coupled with another y.n. has a same digit in a same position, all y.numbers are odd.
???? The explanation is already difficult to understand; but what do these abreviations mean????