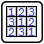
Galaxy Count: Green and Lavender
(Published on 28. January 2024, 21:07 by gdc)
This is a collaboration with Marty Sears. It features circles and zipper lines as well as a ruleset that peaked my interest lately: spiral galaxies. Thanks Nurator for testing, inspiration and feedback. See below for examples for the galaxy types.
- Latin Square: Fill the grid with the digits 1-9. Digits may not repeat in a row or column.
- Fog of War: Enter correct digits to clear the fog. No guessing is necessary.
- Spiral Galaxies: Diamonds in the grid mark the centers of galaxies. Galaxies are a collection of 1 or more orthogonally connected cells which are rotationally symmetrical through 180 degrees around their centers. Every cell in the grid is part of exactly one galaxy, and digits may not repeat in a galaxy. All galaxy centers are given.
- Counting Circles: Each galaxy contains a circle. The digit in that circle is equal to the number of cells in that galaxy. This digit is also equal to the number of circles containing that digit.
- Galaxy Types: Some circles are coloured and have letters attached to them. If present, they define the galaxy type.
- W (whisper galaxy): Orthogonally adjacent digits within a whisper galaxy have a difference of 5 or more.
- Z (zipper galaxy): Each digit and its rotational counterpart in a zipper galaxy sum to the same total. If the galaxy has an odd size, that total equals the digit in the galaxy's center.
Examples
The first image shows an example of a valid whisper galaxy. It acts like a whisper line. In case the galaxy contains 2x2 areas, all adjacent neighbors in the galaxy must differ by 5 or more.
The second image shows a valid zipper galaxy. Digits opposite of the diamond in the center always sum up to 8. If the diamond was in the center of a cell (rather than a corner/edge) the central digit would need to be an 8 as well.
The third image shows an example of a galaxy without a galaxy type.
Solution code: Row 4 (9 digits)
Comments
on 26. September 2024, 22:02 by QuiltyAsCharged
Brilliant puzzle! I don't know how it's 3 star difficulty, maybe that's rounding down from 3.9. I suppose all the steps are fairly simple once you spot them, but spotting them is the real challenge.
on 22. April 2024, 14:15 by lmdemasi
Beautiful puzzle. Great deductions throughout.
on 2. February 2024, 16:30 by jacques_
Absolutely amazing!! Got quite stuck near the end but had a beautiful solve. Thanks so much!!
on 1. February 2024, 01:51 by jacques_
Absolutely amazing!! Got quite stuck near the end but had a beautiful solve. Thanks so much!!
on 31. January 2024, 00:52 by ViKingPrime
There's something about Fog-of-War that attracts some aggressive ratings, isn't there? This is definitely deserving of all the praise, forget the rating.
on 30. January 2024, 04:50 by TheBestThatICouldDo
Wow, this was so fun. This is an incredible use of galaxies. Novel , fresh, and very smooth
on 29. January 2024, 19:27 by Nurator
Awesome puzzle! That deduction Silverscree mentioned is really extraordinary. You have to try this yourself!
on 29. January 2024, 00:59 by Silverscree
That was an excellent combination of constraints. I only felt stuck once, briefly near the start, but that led to a particularly excellent deduction that I won't spoil. Thanks gdc!