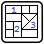
XV Pentominoes
(Published on 28. September 2022, 00:54 by Kayexe)
Created by Kayexe
This is my first puzzle ever published. Feel Free to comment
*I was not able to set the regions properly I think...
Rules:
Every digit from 1 to 9 has to appear once in every row, column and region.
The regions first need to be determined. Every region is a set of nine orthogonally connected cells.
Each Region must fully contain a V or an X Pentomino.
Each Pentomino is made only from the digits 1 to 5
Cells separated by:
- an X sum to 10 and must be from different region
- a V sum to 5 and must be from different region
- a black dot have a 1:2 ratio and must be from different region
- a white dot have a 1:2 ratio and must be from the SAME region
This is an example of a valid solution
This is my first puzzle ever published. Feel Free to comment
Solution code: row 2 followed by column 2 no spaces
Comments
on 12. December 2023, 07:15 by DaleVandermeer
There is a mistake in this puzzle. I guess the dot in the first column is a white dot.
on 13. December 2022, 01:48 by h5663454
THIS IS A WRONG PUZZLE!!!
on 29. September 2022, 14:28 by h5663454
Sorry to bother you, but maybe you should check the puzzle and answer more than clarifying the ruleset.
I solved this puzzle strictly according to the ruleset, but got results that conflict with the ruleset.
on 28. September 2022, 17:06 by meowzzz
Might want to add some clarification to your pentomino rules.
Is the v only a _| as per the example or does it include all orientations |_ _| |¯ ¯| of the shape, does it also include an actual v (non-orthogonally connected)
192
384
657
what about [ ] U ^ type shapes, which are v-like?
Same for the x is it only a + as per the example or does it include and actual x (non-orthogonally connect)
192
837
465
I'm guessing its:
"Each Pentomino is a region of orthogonally connected cells containing the digits 1 to 5"
"Pentomino's form a shape of either an + or L in any rotational orientation"
EDIT: can also turn the "highlight conflicts" off in f-puzzles :)
on 28. September 2022, 15:10 by Kayexe
Added an example