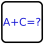Taxicab-kuro
(Published on 20. July 2020, 02:25 by glum_hippo)
Standard Kakuro rules apply. Each cell must contain a digit from 1-9, such that no digit repeats within a 'word' and the digits add up to the clued value.
Also, as an homage to Madmahogany, there is a 'Manhattan distance' or 'anti-taxicab' restriction: if two cells contain the same digit, the taxicab distance (minimum number of orthogonal steps from point A to point B) cannot be equal to that digit.
Example: in the following diagram, due to the givens, 7 is excluded from all blue and green cells, and 4 is excluded from all red and green cells. As one can see, the blackened cells are treated like any other cell in the way the Manhattan distance is calculated.Please note that e.g., the 7 also excludes a 7 that is seven steps directly above it in the column or seven steps directly to the right in the same row! (From Taxicab-Sudoku one is perhaps accustomed to looking for shifts in two perpendicular directions)
And here is the puzzle. The grey cells are just to help with counting and have no influence on the logic. Try it on Penpa!
Solution code: Enter the first row and the last column, ignoring the black cells. (19 digits total; the top right corner cell is entered twice)
Comments
on 21. July 2020, 16:10 by mandourin
This is truly an incredible puzzle. Insanely hard too but so satisfying!
on 21. July 2020, 13:08 by glum_hippo
@Luigi Die 7 ist wegen Kakuro-Regeln von den senkrecht und waagerecht geformten ‘Worten’ ausgeschlossen, und wegen anti-Taxicab-Regeln von den entfernteren Zellen. Die weissen Zellen sind erlaubt weil sie einem anderen Wort angehören UND nicht genau sieben Zellen von der 7 Abstand halten.
on 21. July 2020, 08:51 by Luigi
Ich verstehe das Beispiel nicht. Die 7 ist von allen blau oder grün gefärbten Zellen ausgeschlossen. Bedeutet das, dass sie in den weißen, 4-6 Zellen darüber liegenden Zellen nicht ausgeschlossen ist?