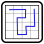
Second Longest Possible Palindrome
(Eingestellt am 11. Juni 2020, 12:48 Uhr von stephane.bura)
If you're looking for the longest possible palindrome, it's in this puzzle
Normal Sudoku rules apply.
The gray line is a palindrome so, for instance, the digit in R2C5 is the same as the one in R3C2.
When the palindrome exits the grid, it continues on the opposite side (from R1C1 to R9C1 and from R4C1 to R4C9).
The dotted rectangles contain digits that add up to 15.
The three dotted circles also contain digits that add up to 15.
Penpa-Edit link for this puzzle to play it online.
Lösungscode: Row 5, column 5
Kommentare
am 8. September 2020, 20:18 Uhr von luda3
Wonderful palindrome sudoku puzzle, thank you for sharing
am 13. Juni 2020, 17:57 Uhr von glum_hippo
@dm_litv 'Endless, senseless flood of pseudo-puzzles' - what a charming comment.
am 13. Juni 2020, 12:33 Uhr von stephane.bura
Changed the title and added the link to the new puzzle.
am 12. Juni 2020, 00:47 Uhr von Madmahogany
Nice one! Very pretty
am 11. Juni 2020, 23:13 Uhr von dm_litv
Ah, sorry. Maybe I was wrong.
But right now I don't see clear prove of the impossibility to make 73.
I have to think about it. At least it's an interesting problem among the endless senseless flood of pseudo-puzzles here recently ;(
am 11. Juni 2020, 20:35 Uhr von Greg
Really enjoyed it.
And now looking forward to the longest palindrome plus 1
am 11. Juni 2020, 20:13 Uhr von stephane.bura
I don't know if it's possible to add a digit in the center of the palindrome. Someone please show me!
Until then, I'll keep this title ;)
am 11. Juni 2020, 19:56 Uhr von stephane.bura
@dm_litv but you're right! There could be one more digit at the center of the palindrome. It was just brain taxing enough to design this with the help of symmetries ;)
am 11. Juni 2020, 19:39 Uhr von dm_litv
Evidently it's not the longest.
am 11. Juni 2020, 14:48 Uhr von KeyserSoze
Enjoyable quick puzzle. Thanks